Abel in Paris

You are welcome to attend Abel in Paris.

Date: Thursday 11. January 2024
Time: 10am – 4pm
Venue: Centre National de la Recherche Scientifique
Hermite Amphitheatre, Institut Henri Poincaré UAR 839 | Sorbonne Université | CNRS
Registration: https://dnva.pameldingssystem.no/abel-in-paris

The event is free, but registration is required.

A light lunch will be served.

Programme

10:00-10:15: Welcome
Sylvie Benzoni, director Institut Henri Poincarè
Lise Øvreås, president the Norwegian Academy of Science and Letters
Helge Holden, chair of the Abel Committee, moderator

10:15-11:15: Yves Meyer

11:30-12:30: Claire Voisin

12:30-13:45: Lunch

13:45-14:45 Nalini Anantharaman

15:00-16:00 Martin Hairer

Lectures

Nalini Anantharaman: On the spectral gap of the laplacian for random hyperbolic surfaces

Abstract:
Although there are several ways to ''choose a compact hyperbolic surface at random'', putting the Weil-Petersson probability measure on the moduli space of hyperbolic surfaces of a given topology is certainly the most natural.

The work of M. Mirzakhani has made possible the study of this probabilistic model: it is one of the only model of ''random riemannian manifolds'' where some explicit calculations are actually possible. One may thus ask questions about of the geometry and the spectral statistics of the laplacian of a randomly chosen surface – in analogy with what is usually asked for models of random graphs.

Nalini Anantharaman will be interested in the spectral gap of the laplacian for a random compact hyperbolic surface, in the limit of large genus (j.w. Laura Monk).

Martin Hairer: Taming infinities

Abstract:
Some physical and mathematical theories have the unfortunate feature that if one takes them at face value, many quantities of interest appear to be infinite! What's worse, this doesn't just happen for some exotic pieces of abstract mathematics, but in the standard theories describing some of the most fundamental aspects of nature. Various techniques, usually going under the common name of "renormalisation" have been developed over the years to address this, allowing mathematicians and physicists to tame these infinities. We will tip our toes into some of the conceptual and mathematical aspects of these techniques and we will see how they have recently been used in probability theory to study equations whose meaning was not even clear until now.

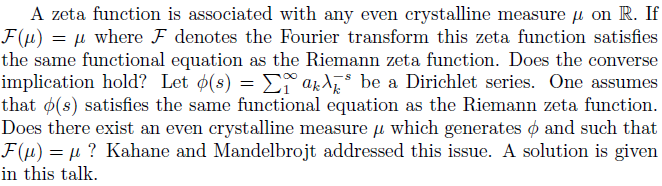
Yves Meyer: Crystalline measures and zeta functions

Abstract:

Claire Voisin: On a problem of Whitney type in algebraic geometry

Abstract:
Whitney embedding theorem in differential geometry says that a small perturbation f' of a map f between two compact manifolds of dimensions m and n (hence f' is isotopic, and a fortiori homotopic to f) is an embedding if n>2m (the Whitney range). I will discuss a related question, asked by Borel and Haefliger, in complex algebraic geometry: can we write the cohomology class of an algebraic subvariety of a smooth projective variety as a combination with integral coefficients of classes of smooth subvarieties? Claire Voisin will discuss classical and recent results on this question, with emphasis on the Whitney range, which has recently been solved in the affirmative by Kollár and herself.

The Abel Prize Committee will also be present:
• Helge Holden (chair)
• Alice Guionnet
• Jun-Muk Hwang
• Martin Hairer
• Ursula Hamenstädt